Cardiac ejection fraction as a problematic metric for heart failure phenotyping

“Seek simplicity and distrust it.”
Alfred North Whitehead (1861–1947), English philosopher and mathematician

The metric ejection fraction (EF) is widely employed to evaluate ventricular pumping performance, used for heart failure (HF) classification, and its calculation is simple.^1–3 For example, with end-systolic volume (ESV) =50 ml and end-diastolic volume (EDV) =100 ml, the EF=50%. Unfortunately, its correct interpretation received insufficient attention. As EF is a ratio-based number (or percentage) without physical unit(s), it cannot provide unique information. The underlying problem, and also a logical solution, can be shown by the ventricular volume domain representation (figure 1A) that relates ESV to EDV. Volumes may be indexed (i) for body surface area, if appropriate. Focusing on ESVi and EDVi is important, as this combination is associated with the familiar pressure–volume (PV) loop description, which, in turn, connects with clinically relevant characteristics, such as stroke work and myocardial oxygen consumption (MVO₂).¹

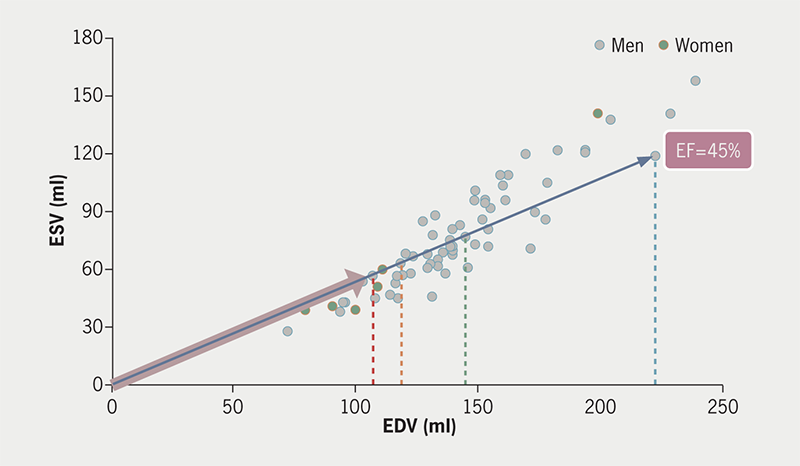
MRI data N=69 (6 women) acute myocardial infarction (AMI) Key: EDV = end-diastolic volume; EF = ejection fraction; EFC = ejection fraction companion; ESV = end-systolic volume

Can paired measurements be converted to yield a single meaningful number?

EF has been adopted as a universally applicable metric and appears to present an optimum corresponding with the sectio aurea.² Despite its popularity,² it remains unclear what precisely constitutes its scientific basis. A recent survey presented pros and cons of EF,³ but failed to identify the major flaw, namely its unitless nature.^4–6 The weakness can be explained by considering analogy with systolic and diastolic arterial blood pressure measurements. Nobody advances the blood pressure ratio (BPR) as a simple measure to define normotension, by a value around 1.5 (for 120 over 80 mmHg – but, perhaps surprisingly, also for 150 over 100, and so on!). Clearly, a BPR of 1.5 may also refer to hypertension, as well as to hypotension, while severity cannot be expressed either. Yet, when referring to ventricular function it is entirely acceptable to speak, e.g. about a worrisome EF of 0.4 (or 40% for that matter). Do we entertain application-specific rules?

EF as paradigm

The clinical relevance of dimensionless ratio-based metrics has been discussed.^4–7 The acclaimed robustness of EF was never established, its use only mildly criticised, but not entirely challenged.⁴ Understanding the real problem underlying the EF paradigm requires appreciation of its mathematical construction. For this purpose, the volume domain representation (ESV vs. EDV) allows preferred exploration, as EF solely depends on both variables.^4,5 This editorial is founded on the insight thus obtained, and describes in plain language the intrinsic limitation of EF.

The diagonal length, given the ratio, defines the size of a TV screen

Mathematically, the EF results from the division of two volumes. This is a familiar algebraic procedure, but somewhat special as the outcome lacks any physical dimension:

Equation 1. EF = (EDV – ESV)/EDV = 1 – (ESV/EDV) = 1 – (ESVi/EDVi)

Importantly, EF depends on the balance between ESVi and EDVi, while both measured volumes can vary over a considerable range.^4,5 Figure 1A displays the two determinants of EF. The calculated EF value is not unique, similarly as earlier shown for BPR. Multiple (matched) {EDV, ESV} combinations generate the same EF, as illustrated for data pairs obtained from 69 acute myocardial infarction (AMI) patients – how could we know which patient’s ventricle we intend to identify from the EF only? All points on any specific line passing from the origin have the same EF, e.g. 45% as shown. This observation emphasises the fundamental shortcoming inherent to the isolated use of EF. The corresponding ESV and EDV values differ, as also reflected by the EF companion (C), which is the distance from the origin to each point, and by the associated distinct PV loops (figure 1B).

Key: EF = ejection fraction; EFC = ejection fraction companion; ESV = end-systolic volume; LV = left ventricle

Indeed, it is impossible to create a single metric (such as EF) that reflects the full information as offered by the underlying two (highly variable) components. Actually, EF requires consideration of an additional (companion) metric, as extensively described elsewhere.⁵ This companion to EF, denoted as EFC, carries physical dimensions (namely ml or ml/m²). While EF remains constant, the EFC reflects the characteristics of the diversity of ESVi and EDVi values corresponding with any fixed EF. This fact is also evident from the current practice to define TV screen size: what matters is the length of the diagonal, given the fixed ratio of height to width. Indeed, the list price for a TV depends on the length of the diagonal (usually expressed in inches). This diagonal is equivalent to the EFC for the ventricle, being calculated in exactly the same manner.⁵

Figure 1C shows in the LV volume domain the {EDVi, ESVi} pairs for 190 HF patients, as described in detail elsewhere.⁸ Here, we specifically address EF in connection with mid-range (mr) phenotyping, flanked by HF with preserved (p) EF (≥50%), and reduced (r) EF (≤40%). For the mr-phenotype we observe an ESVi ranging from 27 to 118 ml/m². Thus, the HFmrEF phenotype refers to a strongly heterogeneous group, despite nearly similar EF values. Again, associated PV-loops are distinct (figure 1D): size, shape and location are all different, with implications for MVO₂.^1,4

Data, courtesy of Professor G R Heyndrickx, Aalst, Belgium8 Reproduced from Kerkhof PL et al. Heterogeneity of patients phenotyped as heart failure with mildly reduced ejection fraction. Eur Heart J, Vol 44, Suppl 2, November 2023, https://doi.org/10.1093/eurheartj/ehad655.825, by permission of Oxford University Press on behalf of European Society of Cardiology. Key: EDVi = end-diastolic volume index; EF = ejection fraction; ESVi = end-systolic volume index; HF = heart failure; HFmrEF = heart failure with a moderately reduced ejection fraction

Key: EF = ejection fraction; ESVi = end-systolic volume index; HFmrEF = heart failure with a moderately reduced ejection fraction; LV = left ventricle

HF ‘ratiotyping’ by using EF

Current HF classification is merely based on the location of the individual {EDVi, ESVi} point, with three options (figure 1C): above the blue line, below the red line, or somewhere between. This recipe for HF phenotyping can be best characterised as ‘ratiotyping’.

Interestingly, it has been documented that EF inversely correlates with the average value of ESVi and EDVi, indicating that EF reflects the mean size of the LV.^8,9 This observation suggests that EF-based phenotyping is a complex alternative route for LV size-based classification.

In summary, HF phenotypes are created by setting cut-off values for the EF ratio, but not for the pivotal EFC. This means that EF-based phenotyping lacks a crucial second criterion. Imagine that, likewise, BPR would be applied to define hypertension and hypotension!

Return to the primary variables

There is a long tradition of associating EF with the severity and prognosis of cardiac disease.^1,2 A low value for EF referred to poor ventricular performance, while values ≥50% belong to normal (i.e. healthy) hearts. Later came a puzzling surprise, with the observation that nearly half of all HF patients do not have EF depression, but rather should be categorised as ‘preserved’, as far as their EF values are concerned.

Theoretically, the limitation inherent to EF can be solved by introducing the EFC.⁵ This route results in a new pair of metrics that is fully equivalent to the original combination of ESVi and EDVi. It can be concluded that there is no need to consider EF, while its application has no advantages either. Therefore, we propose to abandon the EF concept altogether, and instead employ the representation of the familiar ESVi versus EDVi (figures 1A and 1C).

Conclusion and path for the future

As outlined here, application of a simple metric like EF cannot work. EF is an incomplete measure, at best shown to roughly indicate average ventricular volume. Incomplete interpretation leads to the flaw inherent to the EF paradigm. It is preferred to concentrate on the primary data (i.e. the measured ESV and EDV), rather than calculating derived metrics (such as EF). This straightforward approach was successfully applied in the blood pressure domain, and deserves adoption for analysis of ventricular volumetric data.

HF phenotyping, as based on EF, is actually ‘ratiotyping’, resulting in heterogeneous patient groups without connection with the familiar PV-loop representation. The latter has the advantage of offering clinically relevant information in terms of MVO₂, stroke work and ventriculo-arterial coupling.

Conflicts of interest

None declared.

Funding

None.

Editors’ note

This manuscript refers to a presentation at the annual European Society of Cardiology (ESC) congress, held in Amsterdam, on 27 August 2023.

References

1. Kerkhof PLM, Kuznetsova T, Ali R, Handly N. Left ventricular volume analysis as a basic tool to describe cardiac function. Adv Physiol Educ 2018;42:130–9. https://doi.org/10.1152/advan.00140.2017

2. Mihaileanu S, Antohi EL. Revisiting the relationship between left ventricular ejection fraction and ventricular-arterial coupling. ESC Heart Fail 2020;7:2214–22. https://doi.org/10.1002/ehf2.12880

3. Marwick TH. Ejection fraction pros and cons: JACC state-of-the-art review. J Am Coll Cardiol 2018;72:2360–79. https://doi.org/10.1016/j.jacc.2018.08.2162

4. Kerkhof PL, Díaz-Navarro R, Heyndrickx GR, Handly N. La serendipia en torno la fracción de eyección: una revisión de la historia, la casualidad y la cuasi-funcionalidad de una métrica aplaudida [A critical analysis of ejection fraction]. Rev Med Chil 2022;150:232–40. https://doi.org/10.4067/S0034-98872022000200232

5. Kerkhof PLM, Peace RA, Handly N. Ratiology and a complementary class of metrics for cardiovascular investigations. Physiology (Bethesda) 2019;34:250–63. https://doi.org/10.1152/physiol.00056.2018

6. Konradi AO, Maslyansky AL, Kolesova E, Shlyakhto EV, Kerkhof PLM. Role of ratio-based metrics in cardiology. Russ J Cardiol 2020;25:145–53. https://doi.org/10.15829/1560-4071-2020-3929

7. Kerkhof PLM, Antohi EL, Handly N. Sex-specific evaluation of arterial stiffness and left ventricular remodeling: can we rely on dimensionless ratios? JACC Adv 2023;2:100431. https://doi.org/10.1016/j.jacadv.2023.100431

8. Kerkhof PLM, Heyndrickx GR, Diaz-Navarro RA, Antohi EL, Mihaileanu S, Handly N. Ventricular and atrial ejection fractions are associated with mean compartmental cavity volume in cardiac disease. Annu Int Conf IEEE Eng Med Biol Soc 2022;2022:1384–7. https://doi.org/10.1109/EMBC48229.2022.9871315

9. Diaz-Navarro RA, Kerkhof PLM. Left ventricular global function index and the impact of its companion metric. Front Cardiovasc Med 2021;8:695883. https://doi.org/10.3389/fcvm.2021.695883